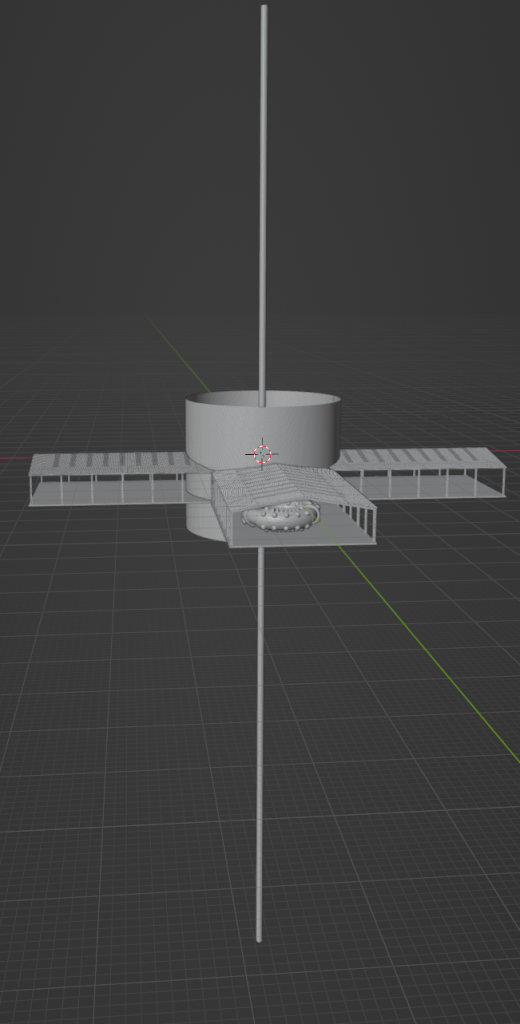
The image below represents a super-gravitational Cavendish-like celestial mechanical pendulum laboratory 1 – 10 kilometers below the surface of the Earth that is currently under construction (in my dreams).

It would be interesting for me to learn how such a hanging mass would move, and how much of the swinging motion (and noise) could be brought to a stop (so as to make further measurements). Perhaps the bottom can be cooled with inlet gases being forced out with a Helium Venturi or some other means.

Dampening every bit of noise is critical, like in all these types of submillimeter-scale gravitational experiments.

The torus shape represents a stellarator that is mass producing neutrons (and Helium).

Neutrons are collected and filtered into a beam, which passes through a small crystal attached to the bottom of a 1-kilogram mass. This suspension is isolated from the environment as much as possible.

Images of neutron diffraction patterns (Bragg’s peaks) are taken to measure tiny displacements.

Perhaps the pendulum fiber may be an ultra-high-molecular-weight polyethylene of no more than .05 millimeters in diameter, and according to calculations, weigh less than 3 grams. Perhaps it can be glass.

Theoretic Displacements

Theoretic displacements at the bottom of the 10 kilometer pendulum would be monitored within the order of 1 angstrom or less, ideally.

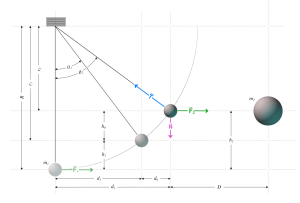
Forces applied, measured, and monitored, would be on the order of magnitude 10^-11 Newtons plus or minus an exponent in units of free-fall acceleration. See the free body diagram below (not drawn to scale), and download the PDF of calculations.

What is the magnitude of kinematic noise that would be interfering with such an experiment 10 kilometers below the surface of the Earth, flying planes, passing trains, and automobiles? And how vibrations can be mitigated, eliminated.

What would the final results of displacements be with respect to the varying degrees of force, as entered into the table. Would there be any surprises?

• PDF of the free body diagram, a short description, a table of values, and static equilibrium calculations.

Related Content

There are some fun videos of Cavendish-like experiments to watch on YouTube, [1] [2]. And a wonderful documentary on LIGO.

NIST has an awesome webpage describing a 2014 workshop on measuring the Newtonian constant G. Several dozen scientists from around the world participated in the gathering.

And there is this March 2023 paper on measuring gravity with milligram levitated masses that I am currently studying.. The gravitational field strength numbers appear to be very close, in the 6 x 10^-10 (m/sec²) range.

Epilogue

After noticing how linear microscopic displacements become with respect to applied forces, I asked myself (see image below): Given that the mass-density of each of the spheres can be anything (e.g., all different), is there a set of such stable displacements that can exist, or almost perfectly linearly?

To me, this looks impossible (the angles are not necessarily to scale). Perhaps as the more linear curvature at the bottom of the radius, the linear approximation becomes increasingly closer until it reaches some quantum state where the spheres are just bouncing around.

Interfaces of tiny displacements in gravitational fields at quantum scales are continually being investigated.